The absorption spectrum of water vapor is not so simple. We have already presented our readers with the absorption spectrum of liquid water. But we cannot simply divide this spectrum by the fraction of water present in air and so obtain the spectrum for water vapor. Water vapor is water dissolved in air. The spectrum of liquid water applies to the Earth's oceans, and to the tiny droplets of water in clouds, but it does not apply to water vapor, as we saw in Vapor Versus Liquid. Water vapor absorbs several orders of magnitude less long-wave radiation than we would expect from dividing the liquid water spectrum by the water vapor concentration.
In liquid water, there are hydrogen bonds between each water molecule and its neighbors. These bonds absorb long-wave radiation. Hydrogen bonds are far less common in water vapor because the molecules are separated. Hydrogen bonds occur in water vapor when pairs of molecules join together to form dimers. The hydrogen bonds in these dimers absorb long-wave radiation. The chance of a water molecule finding a partner to form a dimer increases with concentration. At the same time, the number of water molecules per unit volume increases with concentration. The result is that the absorbing power of water vapor increases as the square of the concentration. Absorption by water vapor is also temperature-dependent because dimers are broken apart by kinetic energy, so colder dimers live longer and have more opportunity to absorb long-wave radiation.
There are also weak long-wave absorbing bonds between water and nitrogen, and water and oxygen. The absorption of long-wave radiation by water vapor is an on-going subject of research at many institutes. The relationship between molecules in water vapor is called the water vapor continuum. In Water vapor absorption coefficients in the 8−13-μm spectral region: a critical review (1990), Grant et al review existing measurements of the water vapor continuum spectrum. In Recent Developments in the Water Vapor Continuum (1999), Mlawer et al review existing calculations of the spectrum. The following graph of water vapor continuum absorption agrees well with measurements such as these.
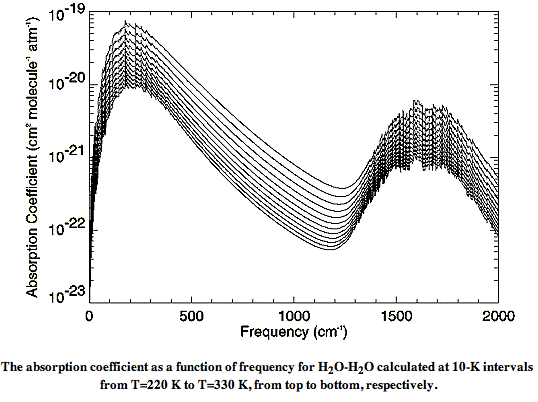
Instead of plotting the spectrum versus wavelength, this graph plots the spectrum versus the inverse of the wavelength, in units of cm−1. The spectrum itself takes the form of a quantity in units of cm2 molecule−1 atm−1, plotted for various temperatures between 220 K and 330 K. In our next post, we will convert this graph into one of absorption length versus wavelength, and show how we can calculate the absorption of long-wave radiation by water vapor in the atmosphere.
PS. Thanks to Peter Newnam for his help researching this post.
UPDATE: See CO2 Continuum for the spectrum of diluted CO2.

A few points on this topic.
ReplyDeleteThe water vapor spectrum actually consists of a line spectrum as well as the continuum spectrum discussed here, and the omission of the discussion of the line spectrum may strike the knowledgeable read as odd. The peak cross sections for the strongest lines are enormous compared with the continuum, so that in a normal atmosphere their optical depth over most of their width is well over one and their effects on transmission are largely in saturation. The lines are also very narrow compared with their separation. So I believe their effects on climate (even counting the many unsaturated weak lines) is negligible for your purposes.
The attribution of the continuum spectrum to dimers apparently is controversial. Similar effects can also be produced by resonance interactions curing collisions between identical molecules without the need of the formation of a dimer. In this model the continuum represents the average effects of the resonance collisions on the tails of the individual lines.
While at low densities the apportionment of the continuum to individual molecules yields an effective cross section that increases with number density, yielding an absorption that is quadratic in density, that is a first order approximation and there is a significant super linear effect at high densities.
At least one of your links (http://www.nersc.gov/news/annual_reports/annrep98/lacis.html) is now broken. It appears that NERSC has removed some of their older annual reports from the web.
Dear William,
ReplyDeleteIf I understand correctly, the formation of dimers is one explanation for the quadratic dependence of absorption upon density, but by no means the only one, and is doubted by some. Furthermore, you say that the continuum absorption is separate from the line absorption, and so the two should be added. We came to this conclusion slowly in writing further posts, but I'm glad to have you state it clearly. Thank you.
In subsequent posts, we turned to the on-line Spectral Calculator, which claims to include both the latest continuum measurements and the line spectra, including line broadening due to proximity of other molecules.
Yours, Kevan