In our carbon cycle equations, MR is the mass of carbon in the oceanic reservoir, MA is the mass of carbon in the atmosphere, kR is the fraction of oceanic reservoir CO2 molecules that will be emitted into the atmosphere each year, and kA is the fraction of atmospheric CO2 molecules that will be absorbed by the oceanic reservoir each year. The rate at which carbon is emitted by the oceanic reservoir is kRMR, and the rate at which it is absorbed by the oceanic reservoir is kAMA. At equilibrium, these two rates will be the same, so we have:
kRMR = kAMA ⇒ MA = kRMR/kA (Eq. 1)
In our previous post we concluded that kA is constant with temperature, while kR increases with temperature as e−2300/T. Our consideration of the Earth's carbon-14 inventory showed that MR = 77,000 Pg. In our natural, equilibrium carbon cycle, we found that MA = 650 Pg. Given that the carbon must reside somewhere, even if MA doubles or halves with temperature, MR will change by less than 1%. So we can assume, to the first approximation, that MR is constant with temperature. It is MA that varies with temperature, and it does so in proportion to kR.
MA = kRMR/kA ∝ e−2300/T (Eq.2)
Aa two temperatures T1 and T2, the equilibrium values of MA, which we denote MA(T1) and MA(T2), are related by:
MA(T2) / MA(T1) = e−2300/T2 / e−2300/T1 = e2300(1/T1−1/T2) (Eq. 3)
Our (Eq. 3) predicts a close, positive correlation between temperature and atmospheric CO2 concentration, and it gives us an estimate of the magnitude of the change in CO2 concentration with temperature. The graph below shows atmospheric CO2 concentration provided by Barnola et al. and global temperature relative to today provided by Petit et al. over the past 425,000 years as determined from the Vostok ice cores.
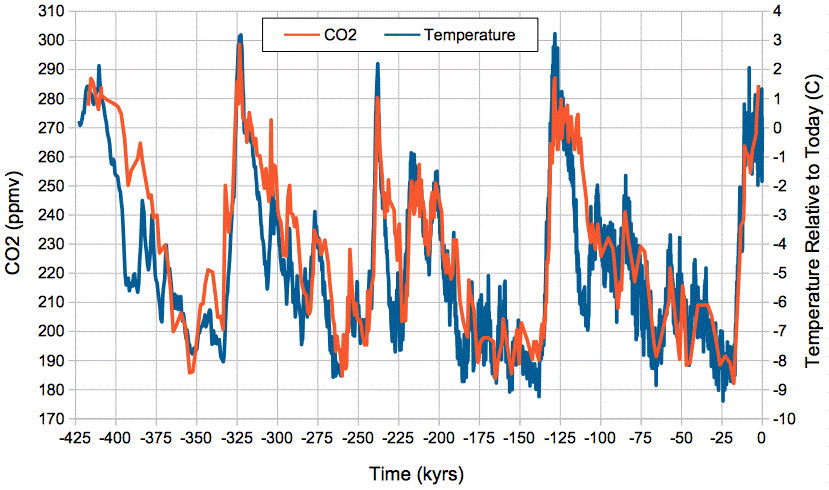
Figure: Absolute Atmospheric CO2 Concentration and Relative Temperature versus Time from Vostok ice cores. Click to enlarge. Local copies of data here and here.
We see close and sustained correlation between CO2 concentration and temperature, even as temperature varies by 12°C. If we assume today's average global temperature is 14°C = 287 K, the change from −9°C to +3°C relative to today is a swing from 278 K to 290 K. Our (Eq. 3) predicts an increase in the mass of carbon in the atmosphere by a factor of e2300(1/278−1/290) = 1.41. Because almost all carbon in the atmosphere is bound up in CO2 molecules, the concentration of CO2 in the atmosphere is proportional to the total mass of carbon in the atmosphere, so when the total mass increases by a factor of 1.41, the CO2 concentration should increase by a factor of 1.41 also. Looking at the graph, we see CO2 rising from 190 ppmv to 290 ppmv, which is a factor of 1.52. Given the many uncertainties in our calculations, and in the ice-core measurements themselves, we are well-satisfied with the agreement between our calculations and the magnitude of the CO2 concentration changes in the ice core measurements.
We conclude that the correlation between CO2 concentration and temperature in the Earth's atmosphere over the past half million years is due to the effect of temperature upon the exchange of CO2 between the atmosphere and the oceanic reservoir of the Earth's carbon cycle. As the temperature rises, the CO2 concentration rises, and when temperature falls, the CO2 concentration falls.
Appendix 1: The van t'Hoff equation for CO2 and water states that the concentration of CO2 above the water is proportional to e−2400/T. Our (Eq. 2) states that it is proportional to e−2300/T. We are well-satisfied with this agreement.
Appendix 2: Suppose we have pure CO2 gas at atmospheric pressure above a reservoir of water. No matter how much CO2 the water dissolves, we maintain the same pressure of CO2 above the water. In this arrangement, unlike the arrangement of our carbon cycle, the concentration of CO2 in the water can vary. Applying the same reasoning we presented in our previous post, the rate at which CO2 molecules are absorbed by the water remains constant with temperature. At equilibrium, the rate at which CO2 molecules are emitted by the water must equal the rate at which they are absorbed, which means the rate of emission must also remain constant. But our calculation states that the probability of any given CO2 molecule in the water being emitted in a certain interval of time must increase with temperature as e−2300/T. If the rate of emission is to remain constant, the concentration of CO2 in the water must decrease with temperature as e2300/T. Only then will the rate of CO2 emission by the water, which is the product of the probability and the concentration, remain constant with temperature. We examine the plot of CO2 solubility in water versus temperature here. As temperature increases from 10°C to 20°C, the solubility of CO2 in water drops from 2.5 g/kg to 1.25 g/kg, which is a factor of 0.50. Our calculation suggests that it should drop by a factor of 0.58. We are well-satisfied with this agreement.

One of our readers is curious about how the natural number, e, keeps cropping up in our calculations. Let's start with the definition of e. We define the factorial of an integer n, denoted n!, to be:
ReplyDeleten! = n × n−1 × n−2 ... ×1
Thus 2! = 2, 3! = 6, 4! = 24. We also define the special cases 1! = 1 and 0! = 1. The natural number, denoted e, is:
e = 1/0! + 1/1! + 1/2! + 1/3! + .. 1/n! + ..
You can perform this calculation yourself, to get the value of e. I went up to n = 10 and arrived at e = 2.718280. If I ask my calcualtor what the exact value of e is, it says 2.718281828.
Kevan,
DeleteWhile your explanation of how to compute e is mathematically sound this calculation does not explain what e actually is.
At school I used to worry where this magic number e came from until I found the following explanation:-
Euler's number e is the point on the X-axis where the area under the curve Y=1/X, from X=1 to X=? is equal to 1. The irrational number e is that point ? on the X-axis that satisfies this area = 1 definition.
Your point is well-taken. I had to abandon the above explanation because the comments would not let me put the symbols I needed into the argument. The explanation you give is also interesting, but does not capture the essence of "e" to my thinking. The number e has the property that the derivative of e^x with respect to x is equal to e^x. That is: the slope of e^x is the same as the value of e^x. For numbers higher than e, the slope is too large, and for numbers lower than e, the slope is too small. In linear systems, we have equations that relate the derivatives of quantities to the quantities themselves, and their higher derivatives as well. In order to have the derivative and the original follow the same shape, the original must vary as e^x or sin(x) or cos(x), but since e^ix (where i is the imaginary number) is equal to cos(x) + i*sin(x), we see that all functions for which the derivatives have the same shape as the original are related to the number e. I like this explanation because I'm an engineer.
DeleteSuppose we have 8,000,000 atoms of carbon-14. One out of every 8000 atoms decays every year. The mass of carbon-14 that decays each year is equal to the total mass of carbon-14 that still exists divided 8000. At first, the carbon-14 decays at a rate of 1,000 atoms per year. But after some time, there will be fewer atoms left, and the decay rate, measured in atoms per year, will be less. When there are only 8000 atoms left, only one will decay every year. The graph of the number of carbon-14 atoms remaining plotted versus time will have this property: its downward slope will be proportional to its height above zero. At the start, the graph has height 8,000,000 and downward slope 1,000 atoms/year. Some time later, the graph has height 4,000,000 and downward slope 500 atoms/year. Far later on, the graph has height 8,000 atoms, and downward slope 1 atom/year. Whatever function of time matches this graph, it has to have this property: its slope is proportional to its value.
ReplyDeleteFurther discourse on this topic is being hampered by the fact that I cannot post HTML wit superscripts to the comments.
ReplyDeleteI am new to this blog and I want to thank you for all the work you put into it. I am spending what free time I have to read and hopefully fully understand it in total. A frustration of mine has been trying to find an open minded and detailed discussion of the AGW theory. In particular, I have been trying to better understand the effects of CO2 vs. water vapor in regards to long wave radiation absorption.
ReplyDeleteI do have a question (my apologies if this was covered already): Has anyone looked at the possibility of increased clouds being a negative feedback on CO2 atmospheric concentration? As clouds increase, so does the absorption of CO2 by the water in the form of carbonic acid?
Welcome Hell_Is_Like_Newark. We have looked hard at the effect of clouds, even building our own simulation that implements snow, rain, heating, reflection, and absorption by CO2. See here for the summary post at the end of our study:
ReplyDeletehttp://homeclimateanalysis.blogspot.com/2012/03/anthropogenic-global-warming.html
As to the beginning of the story, this blog was not pre-planned, but I think you could start here, and skip all subsequent posts on the temperature record.
http://homeclimateanalysis.blogspot.com/2010/01/refutation-of-greenhouse-effect.html
There are many posts about how gas cells behave as they rise. But in the end, you can run our simulation program on your own computer pretty easily, to see for yourself. The answer is Yes, clouds absolutely provide negative feedback.
We have not looked at the absorption of CO2 by clouds. I had not thought of that mechanism before. I just assumed the CO2 was being dissolved at the ocean surface. But the microscopic water droplets in clouds have a vast surface area for dissolving CO2, and it could be that they are the dominant means by which CO2 ends up in the ocean. Thank you for that thought. We'll try to look into it later in this series.
ReplyDeleteThe carbonic acid formation was something I remembered from my grade school earth science classes.
DeleteI have wondered how concentration of carbonic acid affects cloud emissivity and reflective properties. For example, when Mt. Pinatubo has it VEI 6 eruption, it blasted enough SOx into the upper atmosphere than when mixed with water created clouds that reflected enough sunlight back out into space to reduce global temps by about 0.5 oC. I remember a couple cool summers and some great skiing for a few years after. Would carbonic acid have a similar or opposite effects?
My background is in mechanical engineering / energy systems. I work a company that specializes in reducing energy consumption for our clients. However, I see a lot of money and effort spent on reducing 'carbon footprints'.. often IMHO, what measures instituted to do so are a vast wast of money and other resources. Hence my interest in wanting to understand what is really going on in much greater detail.
Clouds of pure water are close to perfect absorbers and radiators of wavelengths 2-20 um, which are the wavelengths radiated by objects at 200-400 K. At the same time, thick clouds reflect 90% of incoming sunlight. It could be that SOx catalyzes the creation of clouds, but I don't think the SOx clouds would be more reflective than pure-water clouds. Thank you for description of your background. So far, we have not found evidence that carbon emissions are warming the Earth, nor that they are likely to cause significant warming. At today's emission rate, it will take 6,000 years to double the atmospheric CO2 concentration, so it looks like the entire concern, although well-intentioned, was off by a couple of orders of magnitude.
ReplyDeleteFrom my understanding the SOx reacts with water to form a sulfuric acid aerosol. If the volcano erupts with enough force, it forces the SOx to the stratosphere, where it stays a lot longer than if the SOx was deposited in the troposphere.
DeleteThis is what I could find in regards to experiments to determine the reflective properties of sulfuric acid aerosols:
https://www.cfa.harvard.edu/HITRAN/HITRAN2012/Aerosols/papers/palmer_williams_h2so4_1975.pdf
With CO2, if the atmosphere warms does the absorption of C02 into clouds (forming carbonic acid) rate increase? If so, that would be another negative feedback.
I see, so SOx itself reflects sunlight. Interesting. I could not get that link to work, not sure why, it looks okay. As to CO2 into clouds, no, the rate of dissolving into water does not increase with temperature, see Effect of Temperature.
ReplyDeleteDear Kevan, good to see that you have been pursuing a new line of investigation. I'll see if I can follow your argument. Regards, Hugh Roper
ReplyDeleteWelcome back, Hugh. The carbon cycle investigation begins back in Carbon-14: Origins and Reservoir. I will welcome your comments.
ReplyDelete